proofsareart - Tumblr blog

proofsareart · 8 years

Photo

Combinatorics. Combinatorics was classically considered the theory of systematically counting highly structured collections of objects, but the word has since broadened to mean essentially any mathematics dealing primarily with finite objects.

This proof shows a relationship between the theory of graphs, which are dots with lines connecting them, and arrangements of high-dimensional flat spaces (analogous to points, lines, and planes) called hyperplanes. The regions of space cut out by the hyperplanes tell us precisely how we may draw arrows on the lines of a graph in such a way that by starting anywhere and following arrows, we never return to our starting location.

#math #maths #mathematics #mathema #combinatorics #graph theory #hyperplane arrangements #myproof #notmytheorem

25 notes · View notes

proofsareart · 8 years

Photo

Order Theory. Order Theory is the study of generalized notions of inequality for numbers. In particular, we are often interested in cases where we only have a “partial” ordering: you might have some best friends, who are better than all the non-best friends, but there’s no way you could ever pick one “bestest” friend!

The motivation for this post is that the word Nötherian (pronounced neu-THEER-ee-in) come up a lot of places and means somewhat different things; this is an unfortunate place where they overlap. However, one thing that it has in common across its many guises: if any object is called Nötherian, you can be sure that there is something finite about the structure of its subobjects.

#math #maths #mathematics #mathema #order theory #topology #myproof #mytheorem

44 notes · View notes

proofsareart · 8 years

Photo

Algebraic Combinatorics. Combinatorics is the systematic study of counting structured collections objects. Algebraic combinatorics is doing this, but with a special emphasis on using notions of symmetry and linearity to help with the counting process. A technique that is often indicative of algebraic combinatorics (although by no means exclusive to this field) is to use lists of numbers called “generating functions” as a way of solving many similar counting problems simultaneously. Remarkably, it is often easier to proceed in this way than to focus attention on a single problem!

Students of calculus may recognize this theorem as the dreaded “partial fraction decomposition”. This trick can be used to make tedious calculus problems, but it has a less unseemly application: the most serious step in the proof that all rational functions have elementary antiderivatives.

#math #maths #mathematics #mathema #algebra #calculus #linear algebra #myproof #notmytheorem

71 notes · View notes

proofsareart · 8 years

Photo

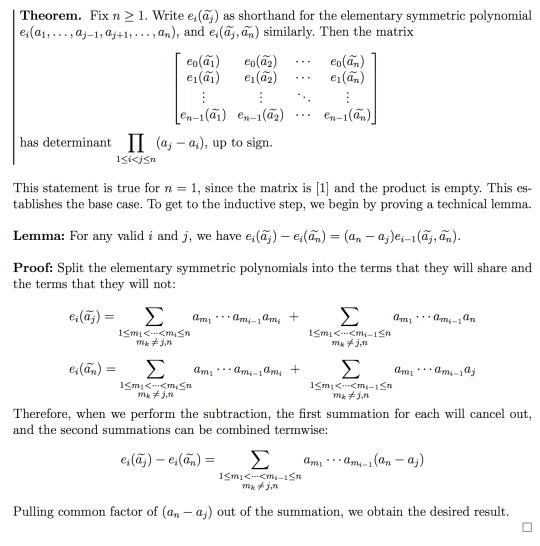
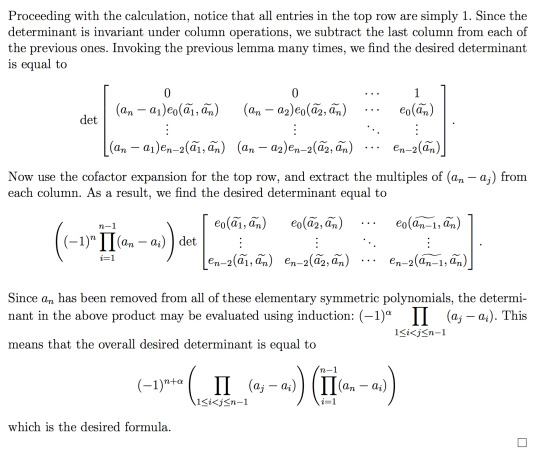
Algebraic Combinatorics. Combinatorics is the systematic study of counting structured collections objects. Algebraic combinatorics is doing this, but with a special emphasis on using notions of symmetry and linearity to help with the counting process.

A very large chunk of algebraic combinatorics— including this theorem— is concerned with things called symmetric functions. In general, a (multivariate) function is a rule that takes in a list of numbers and gives out a new number. But a symmetric function is “nicer” in that it only cares about which numbers you give it, and not the order that those numbers are in.

This is probably a very well-known result, but I was very pleased to have discovered it myself. I didn’t come up with it out of thin air, though; I had a very specific application in mind, which will be the subject of the next post.

#math #maths #mathematics #mathema #combinatorics #algebraic combinatorics #algebra #linear algebra #matrices #myproof #mytheorem

51 notes · View notes

proofsareart · 8 years

Photo

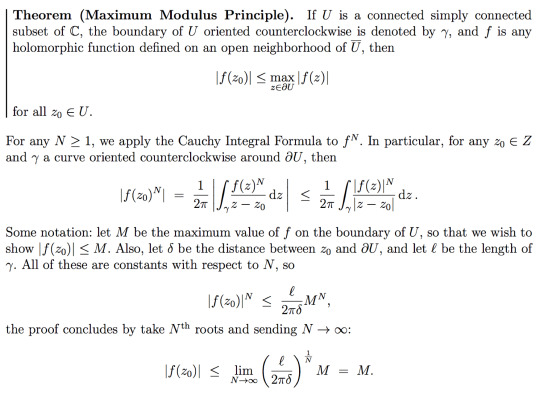
Complex Analysis. Analysis is the study of functions, or “maps”, that are rules for transforming objects into other objects; complex analysis is interested in those maps for which the original objects are complex numbers. Such maps have an incredible amount of structure, and there are many pathological behaviors that we would expect from such maps which, to our delight, are ruled out by this structure.

The curly “d” is pronounced “boundary", and its technical meaning is a formalization of the ordinary-language meaning of the word boundary.

I wish I could take credit for this proof, but it is not mine. I learned it from the professor for a graduate course on analytic number theory.

#math #maths #mathematics #mathema #analysis #complex analysis #notmytheorem #notmyproof

33 notes · View notes

proofsareart · 9 years

Photo

Algebra. Algebra is the deep study of operations like multiplication, which turn two objects into a single object. This proof lies in the area of ring theory, which is concerned with the interaction of two such operations whose interaction is analogous to that of addition and multiplication. However, the “addition” and “multiplication” operations themselves are allowed to be rather exotic. For instance, ordinary multiplication has infinitely many “atomic” elements called prime numbers, but this proof discusses what happens when the multiplication admits just a single prime!

#math #maths #mathematics #mathema #algebra #abstract algebra #ring theory #myproof #notmytheorem

33 notes · View notes

proofsareart · 9 years

Photo

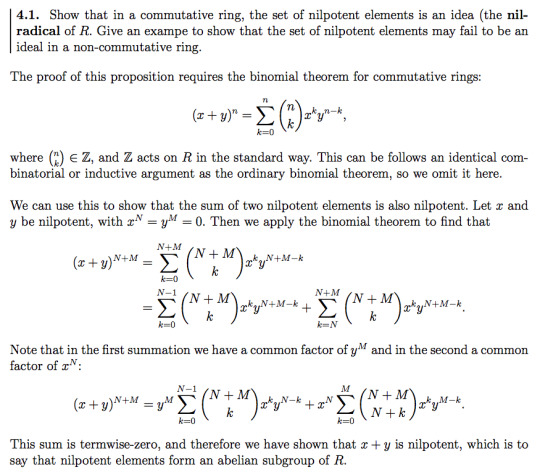
Algebra. Algebra is the deep study of operations like multiplication, which turn two objects into a single object. This proof lies in the area of ring theory, which is concerned with the interaction of two such operations whose interaction is analogous to that of addition and multiplication. However, the "addition” and “multiplication” operations themselves are allowed to be rather exotic. For instance, this proof discusses a basic property of the “nilradical”. This is the set of nonzero elements, which have the counterintuitive property that, when multiplied by themself enough times, they give an answer of zero!

#math #maths #mathematics #mathema #algebra #abstract algebra #ring theory #myproof #notmytheorem

82 notes · View notes

proofsareart · 9 years

Photo

Combinatorics. Combinatorics is, nowadays, a “catch all” adjective that describes a wide variety of mathematical fields. However, it began with what is today called “enumerative combinatorics”, the systematic study of counting objects. This proof is a generalization of a celebrated result called the binomial theorem, which relates repeated multiplication to the numbers in the famous Pascal’s triangle.

Included in the proof is a (literal) sketch of the main idea, which is a certain correspondence: sequences of white and black boxes, on one hand, and arrangements uncolored boxes into a bunch of rows that get smaller as you go down the page.

#math #maths #mathematics #mathema #combinatorics #partitions #partition theory #generatingfunction #binomial coefficient #pictures #myproof #notmytheorem

52 notes · View notes

proofsareart · 9 years

Photo

Functions. Functions are extremely important mathematical objects which dictate rules for turning objects of one kind into objects of another kind. They are ubiquitous in mathematics and are so critical to almost every field that I believe them to be the central objects of study in mathematics. One of the things that makes functions so significant is that the rules can be performed in succession to produce a new rule; this operation is called composition and it provides a useful structure on the class of functions.

#math #maths #mathematics #mathema #functions #set theory #myproof #mytheorem

53 notes · View notes

proofsareart · 9 years

Photo

Combinatorics. Combinatorics is an extremely broad subject that covers nearly all of finite math. The term originally referred to a discipline which is not called enumerative combinatorics, which is the study of counting (finitely many objects). One of the interesting features about combinatorics is a certain method of proof that it invites, where due to the physical-like nature of the objects being manipulated, can be described with very little symbol pushing. In fact, combinatorialists often prefer these types of proofs because they often better convey intuition about why a result is true, than can their algebraic counterparts.

#math #maths #mathematics #mathema #combinatorics #Enumerative combinatorics #binomial coefficient #bijective proof #low symbol count #myproof #notmytheorem

62 notes · View notes

proofsareart · 9 years

Photo

Induction. Induction is a method usually used to prove statements regarding natural numbers when one only has information about the "local" truth of these statements. It is the formal mechanism underlying recursion, which is commonly used in (functional) computer programming as a way to do similar tasks over and over again.

Induction can sometimes be considered a little inelegant, but it is often an essential feature lying in the background of beautiful work.

#math #maths #mathematics #mathema #induction #number theory #numbers #base #digits #modular arithmetic #myproof #mytheorem

27 notes · View notes

proofsareart · 9 years

Photo

Transcendental Number Theory. A number is called “algebraic” if it is the root of some polynomial, and it is called “transcendental” if it is not. Algebraic numbers can be thought of as a generalization of rational numbers (fractions) which allow some additional flexibility. Algebraic numbers have long been known to be in the vast minority among the real numbers, but it turns out that if I hand you a real number, it is generally very difficult to determine if it is transcendental.

It is known, for instance, that π and e are transcendental, but the usual proof of these facts uses very intense machinery.

(This is another one of those awkward "who gets credit” situations: the proof, I think, is a fairly straightforward augmentation of Liouville’s classic proof, but I don’t think he ever formulated it in these terms. In any case, it is not such a significant extension that it was likely to be published. In any case, at this level of generality it was first brought to my attention by fellow student Louie Corwin.)

#math #maths #mathematics #mathema #number-theory #transcendental #algebraic #transcendental number theory #analytic number theory #notmyproof #notmytheorem

68 notes · View notes

proofsareart · 9 years

Photo

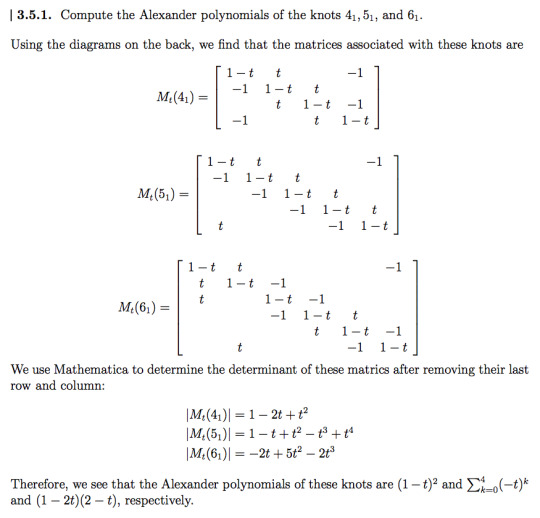
Knot Theory. Knot theory is the study of how circles can be embedded into three-dimensional space. It is like ordinary knot that we might tie in a rope, except after we tie it we must fuse the ends of the rope together. Originally founded as an attempt to understand molecular structure (in a model requiring the now-defunct notion of aether), knots find application today in the study on larger chemicals, for instance the macrostructure of DNA.

It turns out that while it is not too hard (theoretically) to show that two knots are the same, proving that two knots are different directly from the definition is an insurmountable task, since one might be really "tangled". Some of the earliest and most successful ways to tell knots apart is to assign to them lists of numbers, such as the "Alexander polynomial" and guarantee that if the lists are different, then the knots are different. (However, if the lists are the same, the knots might still be different: it doesn't do all your work for you).

[[The diagrams on the back are presented above the post, with admittedly less detail than you need to actually produce the calculations.]]

I must apologize again for the dearth of posts recently; thanks to my work with the Collaborative Research Project, I have even less free time than usual to devote to blogging :( I'm not going to make any promises, but I will say that spring break is around the corner— as well as the only pi day of our lives (which we can still appreciate even though pi is wrong).

#math #maths #mathematics #mathema #knot theory #topology #knot #polynomials #pi day is coming #tau #notmytheorem #myproof

95 notes · View notes

proofsareart · 9 years

Photo

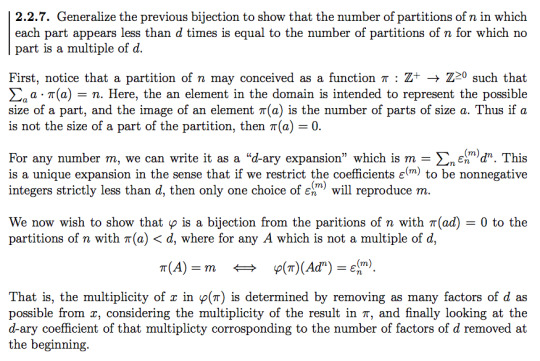
Combinatorics. Combinatorics began as the systematic study of counting the number of elements in particularly common sets. Nowadays, this definition has broadened and you can use “combinatorics” to refer to pretty much all of finite mathematics. The original field is now more descriptively called “enumerative combinatorics”.

This proof concerns two subclasses of objects called partitions, which are simply (unordered) lists of positive numbers with some fixed sum. For instance, 5 has five partitions: 3+2, 3+1+1, 2+2+1, 2+1+1+1, and 1+1+1+1+1.

#math #maths #mathematics #mathema #combinatorics #enumerative combinatorics #partitions #partition theory #myproof #notmytheorem

63 notes · View notes

proofsareart · 9 years

Photo

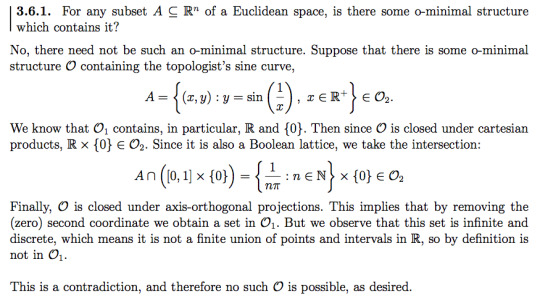
Descriptive Set Theory. Set Theory is the formal study of sets, the objects which mathematicians use in order to speak about multiple objects at once. Descriptive Set Theory restricts the discussion to sets which are in some precise sense "tractable". Unfortunately, there are many natural features which might allow or preclude a set from being tractable, and they do not play nicely with one another: if you allow all tractable sets, the resulting collection is too varied to easily reason with. One of the classical goals of DST was to try to determine which collection was most useful.

An o-minimal structure is a (graded) collection of sets which one can think of as being "geometric". This is not the most commonly used formalization of this intuition, and the two are not consistent. However, it is useful as the setting in which we can step back and forth between the worlds of topology (study of shapes and dimension) and combinatorics (counting techniques).

I formulated this question during my attempt to understand a free textbook I am reading called Elementary Applied Topology, which unfortunately does not contain any exercises. I am writing more about my experience reading this book over at OTAM (my new blog).

#math #maths #mathematics #mathema #set theory #descriptive set theory #dst #geometry #mytheorem #myproof

62 notes · View notes

proofsareart · 9 years

Text

New Years' Resolution

Hello, everyone!

This post is, first and foremost, an apology to all of my wonderful followers, who have been the driving creative force behind about 90% of this blog's history, who have been nothing but supportive and welcoming, who I did not consider when I kept pushing back a hiatus announcement "one more weekend", and who have transformed this once-throwaway experiment into one of the more meaningful aspects of my life.

I have excuses for my behavior, but I have no compelling reasons. I am sorry.

I hope that you will find it in your heart to forgive my careless neglect and return with me as we travel these mathematical worlds and find the beautiful creatures which we call proofs.

------

This post is also an advertisement, because nothing says "I'm sorry" like advertising? I'm making a resolution this New Years, and as a result I am going to be posting on this blog again, and also starting another one. For an explanation of why this is happening and what it means, check out the first post at the new blog, One Thousand Adventures in Mathematics. [thousandmaths.tumblr.com]

#sorry #apology #followers #so much love #advertisement #whiplash?#wasn't sure where to put the apostrophe #you'll understand if you read the OTAM post #first world problems

4 notes · View notes

proofsareart · 10 years

Photo

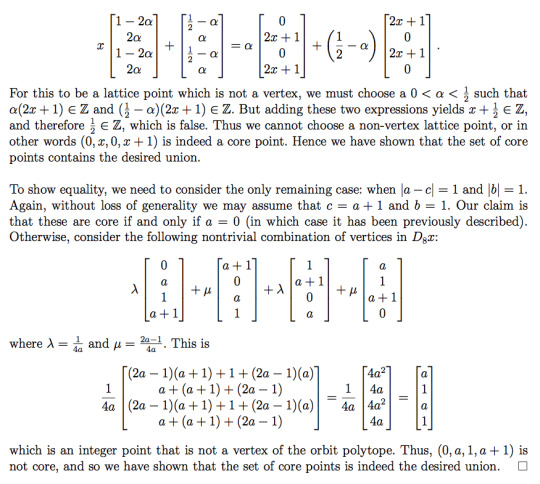
Operations Research. OR is the study of optimization problems, and one of the central methods used is called integer programming. Unfortunately, the method is generally very difficult to solve, but in many special cases it turns out to be tractable.

I'm sorry that I have not been posting much. Research has not been moving quickly at all, and while I've always "known" that this is pretty much how research goes, experiencing it firsthand for the first time has been really emotionally draining. There were other extenuating circumstances of course, because life doesn't hand you problems one at a time, but I think this ended up being the big one.

Now, I'm learning to take it a bit easier, and I'm actively working to get my ego out of the fucking game. In any case, I think I'm stronger for the experience, and if nothing else it's probably good training for my thesis :D

#math #maths #mathematics #mathema #operations research #optimization #integer programming #research #day9 is giving me life advice again #mytheorem #myproof

79 notes · View notes